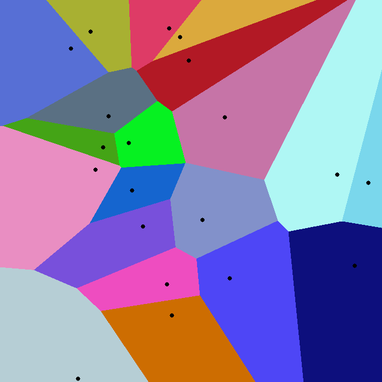
In mathematics, a Voronoi diagram is a way of dividing space into a number of regions. A set of points (called seeds, sites, or generators) is specified beforehand and for each seed there will be a corresponding region consisting of all points closer to that seed than to any other. The regions are called Voronoi cells. It is dual to the Delaunay triangulation.
It is named after Georgy Voronoy, and is also called a Voronoi tessellation, a Voronoi decomposition, a Voronoi partition, or a Dirichlet tessellation (after Peter Gustav Lejeune Dirichlet). Voronoi diagrams can be found in a large number of fields in science and technology, even in art, and they have found numerous practical and theoretical applications.
Follow the link below to see an animated Voronoi Tessellation … be the wandering point …
The Voronoi tessellation shows the closest point on the plane for a given set of points. This example updates the Voronoi diagram in response to mouse interaction! Colors by Cynthia Brewer; algorithm by Steven Fortune; implementation based on work by Nicolas Garcia Belmonte; interaction inspired by Raymond Hill.
Also have a look on the base javascript library … d3js.org